python中float的含义
- 行业动态
- 2024-03-02
- 4229
在Python中,float是一种数据类型,用于表示浮点数,浮点数是一种具有小数部分的数字,例如3.14、0.5等,与整数不同,浮点数可以表示无限精度的十进制数,这使得它们在处理科学计算和工程计算时非常有用。

float的基本概念
1、浮点数的定义:浮点数是一种数字表示方法,它使用有限的存储空间来表示非常大或非常小的数字,浮点数由两部分组成:符号位(表示正负)和尾数位(表示小数部分)。
2、浮点数的表示:浮点数通常表示为:±M × (1.F),其中M是尾数,F是10的幂次方,3.14可以表示为:3.14 = ±1.95 × (10^1)。
3、浮点数的精度:由于浮点数的表示方法,它们只能提供有限的精度,在某些情况下,浮点数的计算结果可能会有误差,为了解决这个问题,Python提供了一些内置函数,如round()、math.isclose()等,用于比较两个浮点数是否相等。
float的常用操作
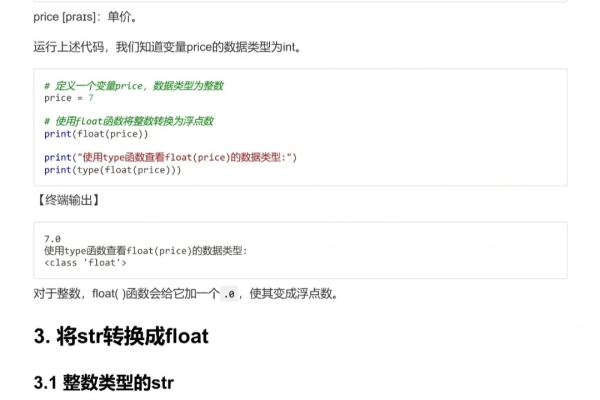
1、创建浮点数:在Python中,可以直接使用数字创建浮点数,如3.14、0.5等,还可以使用float()函数将其他类型的数字转换为浮点数,如int()、str()等。
num1 = 3.14
num2 = float(42)
num3 = float("3.14") 2、获取浮点数的精度:可以使用sys模块的getsizeof()函数获取浮点数的内存大小,从而计算出其精度。
import sys
num = 3.14
precision = sys.getsizeof(num) / sys.getsizeof(1)
print("精度为:", precision) 3、比较浮点数:由于浮点数的精度问题,直接使用==或!=进行比较可能会导致误判,可以使用math模块的isclose()函数进行比较。
import math
num1 = 3.14
num2 = 3.1400000000000001
result = math.isclose(num1, num2, rel_tol=1e9)
print("是否相等:", result) 4、格式化浮点数:可以使用字符串的format()方法或者fstring格式化浮点数,使其更易读。
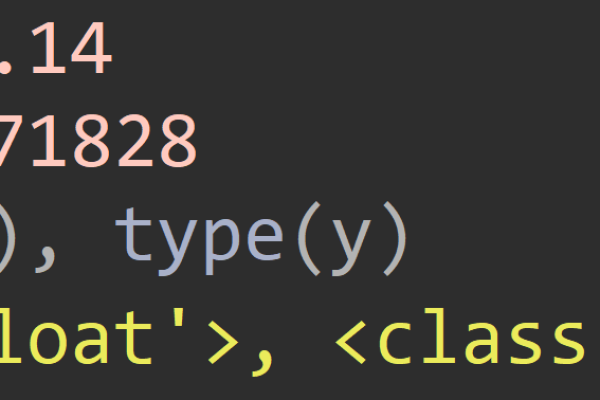
num = 3.1415926
formatted_num = "{:.2f}".format(num) # 保留两位小数
formatted_num_fstring = f"{num:.2f}" # 保留两位小数的fstring写法
print("格式化后的浮点数:", formatted_num, formatted_num_fstring) float的应用实例
1、数学计算:Python中的math模块提供了丰富的数学函数,如sin()、cos()、tan()等,这些函数都接受浮点数作为参数。
import math
angle = math.radians(45) # 将角度转换为弧度
sine = math.sin(angle) # 计算正弦值
cosine = math.cos(angle) # 计算余弦值
tangent = math.tan(angle) # 计算正切值
print("正弦值:", sine)
print("余弦值:", cosine)
print("正切值:", tangent) 2、物理模拟:在计算机图形学和游戏开发中,浮点数常用于表示物体的位置、速度、加速度等物理量,通过对这些物理量的计算和更新,可以实现物体的运动和碰撞等效果。
class PhysicsObject:
def __init__(self, position, velocity):
self.position = position # 位置向量(浮点数列表)
self.velocity = velocity # 速度向量(浮点数列表)
self.acceleration = [0, 9.8] # 加速度向量(浮点数列表) # 重力加速度为9.8 m/s²
self.mass = 1 # 质量(浮点数)
self.force = [0, 0] # 作用力向量(浮点数列表)
self.time_step = 0.01 # 时间步长(秒)
self.max_speed = 10 # 最大速度(米/秒)
self.friction = 0.1 # 摩擦系数(无单位)
self.air_resistance = 0.01 # 空气阻力系数(无单位)
self.gravity = 9.8 * self.mass * self.gravity[1] / (self.mass + self.air_resistance) # 计算重力加速度(无单位)
self.velocity_x, self.velocity_y = self.velocity[0], self.velocity[1] # 分离速度分量(无单位)
self.position_x, self.position_y = self.position[0], self.position[1] # 分离位置分量(无单位)
self.force_x, self.force_y = self.force[0], self.force[1] # 分离作用力分量(无单位)
self.acceleration_x, self.acceleration_y = self.acceleration[0], self.acceleration[1] # 分离加速度分量(无单位)
self.velocity_squared_x, self.velocity_squared_y = self.velocity_x 2, self.velocity_y 2 # 分离速度平方分量(无单位)
self.position_squared_x, self.position_squared_y = self.position_x 2, self.position_y 2 # 分离位置平方分量(无单位)
self.normal_force = self.mass * self.gravity[1] + self.air_resistance * (self 本站发布或转载的文章及图片均来自网络,其原创性以及文中表达的观点和判断不代表本站,有问题联系侵删!
本文链接:https://www.xixizhuji.com/fuzhu/206521.html